HOOKE’S LAW

HOOKE’S LAW; MODULUS OF ELASTICITY BASIC INFORMATION

What Is Hooke's Law? How To Apply Hooke's Law?

Most engineering structures are designed to undergo relatively small deformations, involving  the straight-line portion of the corresponding stress-strain diagram. For that initial portion of the diagram, the stress s is directly proportional to the strain P, and we can write


σ = ΞE

This relation is known as Hooke’s law, after Robert Hooke (1635–1703), an English scientist and one of the early founders of applied mechanics. The coefficient E is called the modulus of elasticity of the material involved, or also Young’s modulus, after the English scientist Thomas Young (1773–1829).

Since the strain P is a dimensionless quantity, the modulus E is expressed in the same units as the stress s, namely in pascals or one of its multiples if SI units are used, and in psi or ksi if U.S. customary units are used.

The largest value of  stress for which Hooke’s law can be used for a given material is known as proportional limit of that material. In the case of ductile materials possessing a well-defined yield poin, the proportional limit almost coincides with the yield point.

For other materials, the proportional limit cannot be defined as easily, since it is difficult to determine with accuracy the value of the 63 stress s for which the relation between s and P ceases to be linear. But from this very difficulty we can conclude for such materials that using Hooke’s law for values of the stress slightly larger than the actual proportional limit will not result in any significant error.

Some of the physical properties of structural metals, such as strength, ductility, and corrosion resistance, can be greatly affected by alloying, heat treatment, and the manufacturing process used. For example, we note in the stress-strain diagrams of pure iron and of three different grades of steel that large variations in the yield strength, ultimate strength, and final strain (ductility) exist among these four metals.

HOOKE’S LAW

           Hooke's Law

All of them, however, possess the same modulus of elasticity; in other words, their “stiffness,” or ability to resist a deformation within the linear range, is the same. Therefore, if one high-strength steel is substituted for a lower-strength steel in a given structure, and if all dimensions are kept the same, the structure will have an increased load-carrying capacity, but its stiffness will remain unchanged.

For each of the materials considered so far, the relation between normal stress and normal strain, s 5 EP, is independent of the direction of loading. This is because the mechanical properties of everyone material, including its modulus of elasticity E, are independent of the direction considered. Such materials are said to be isotropic.

Hooke's Law

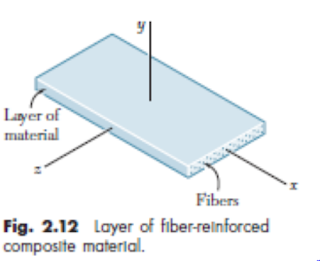
Materials whose properties depend upon the direction considered are said to be anisotropic. An important class of anisotropic materials consists of fiberreinforced composite materials. These composite materials are obtained by embedding fibers of a strong, stiff material into a weaker, softer material, referred to as a matrix.

Typical materials used as fibers are graphite, glass, and polymers, while various types of resins are used as a matrix. Figure 2.12 shows a layer, or lamina, of a composite material consisting of a large number of parallel fibers embedded in a matrix.

            Hooke's Law

An axial load applied to the lamina along the x axis, that is, in a direction parallel to the fibers, will create a normal stress sx in the lamina and a corresponding normal strain Px which will satisfy Hooke’s law as the load is increased and as long as the elastic limit of the lamina is not exceeded.

Similarly, an axial load applied along the y axis, that is, in a direction perpendicular to the lamina, will create a normal stress sy and a normal strain Py satisfying Hooke’s law, and an axial load applied along the z axis will create a normal stress sz and a normal strain Pz which again satisfy Hooke’s law.

However, the moduli of elasticity Ex, Ey, and Ez corresponding, respectively, to each of the above loadings will be different. Because the fibers are parallel to the x axis, the lamina will offer a much stronger resistance to a loading directed along the x axis than to a loading directed along the y or z axis, and Ex will be much
larger than either Ey or Ez.

A flat laminate is obtained by superposing a number of layers or laminas. If the laminate is to be subjected only to an axial load causing tension, the fibers in all layers should have the same orientation as the load in order to obtain the greatest possible strength. But if the laminate may be in compression, the matrix material may not be sufficiently strong to prevent the fibers from kinking or buckling.

The lateral stability of the laminate may then be increased by positioning some of the layers so that their fibers will be perpendicular to the load. Positioning some layers so that their fibers are oriented at 308,
45 deg, or 60 deg to the load may also be used to increase the resistance of the laminate to in-plane shear.

Hooke's Law

Go home page - https://kdlearners.blogspot.com-

http://eunsetee.com/Y0zx